[an error occurred while processing this directive]

Home > College of Sciences > Institute of Fundamental Sciences > Maths First > Online Maths Help > Calculus > Differentiation > Tangents, Derivatives and Differentiation > The Slope of a Tangent Line	SEARCH MASSEY
	LIBRARY \| NEWS \| EVENTS

[an error occurred while processing this directive]

Tangents, Derivatives and Differentiation

The Slope of a Tangent Line

Graphical representation of a phenomenon provides a very useful visual interpretation of that phenomenon. One very important feature of any graph is whether it is rising (increasing) or falling (decreasing). The rise and fall, peaks and troughs of a graph can be studied using the tangent lines to the graph.

If a tangent has a positive slope the graph is increasing around the point of tangency. If a tangent has a negative slope the graph is decreasing around the point of tangency. If a tangent is horizontal the graph is often, but not always, at a peak or trough at the point of tangency.

We are interested in a graph that is determined by a function. But how can one obtain a tangent line, and therefore its slope without drawing a graph? We can calculate one approximately as follows:
Suppose there is a tangent line at a point P = (x, y) on the graph of a function y = f(x). We take another point near P, say Q = (x+h, f(x+h)).

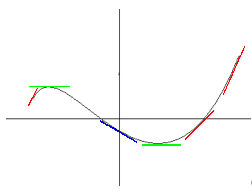
As we move Q closer to P (i.e. let h approach zero), the secant line PQ gets closer to the tangent line at P and the slope of PQ gets closer to the slope of the tangent.

Now the slope of the secant line PQ is

This is known as a Newton’s quotient.

As h gets nearer to zero (we write ), the above quotient gives the slope of the tangent. We write this as

and read this as the “limit of Newton’s quotient as h tends to 0”.


Tangent and Secant Lines

Launch the tangent applet provided below to investigate the connection between the slope of the secant and the tangent for the curves given, or enter equations of your own curves in the box provided.
Drag the red point to see how the tangent changes along the curve. Drag the green point to see how the secant line approaches the tangent as the green dot (Q in our discussion above) approaches the red dot (P in our discussion above). Not also how each of the slopes of the tangent and secant lines change (shown in the box in the upper left hand corner).

As the secant line approaches the tangent line, the slope of the secant line gets closer to the slope of the tangent.

Can't see the above java applet? Click here to see how to enable Java on your web browser. (This applet is based on free Java applets from JavaMath )

<< The Slope of a Line | Differentiation Index | The Derivative >>