Increasing,
Decreasing, Stationary
Let f(x) be a function and assume that for each value of x, we can
calculate the slope of the tangent to the graph
y = f(x) at x. This slope depends on the value of x that we choose, and so is itself a function. We call
this function the derivative of f(x) and denote it by f ´
(x).
| |
The
derivative
of f(x) at the point x is equal to the
slope of the tangent
to y = f(x) at x.
|
|
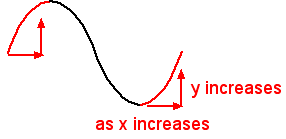
The graph of a function y = f(x) in an interval is increasing
(or rising) if all of its tangents have positive slopes. That is, it is increasing if
as x increases, y also increases.

The graph of a function y = f(x) in an interval is decreasing
(or falling) if all of its tangents have negative slopes.
That is, it is decreasing if as x increases, y decreases.

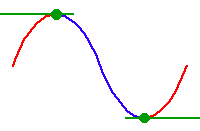
The graph of a function y = f(x) has a stationary
point at the point where the tangent is horizontal
or has zero slope. This always
occurs at the points where a function changes from increasing to
decreasing and at the points where a function changes from decreasing
to increasing. It can also occur at other points and we will discuss
this possibility later.
When the graph of a function y = f(x) is vertical or
discontinuous the tangent is undefined.
These basic properties of the derivative are summarized in the
following table.
Example
Let 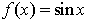 . Then . Then 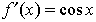 . .
Since 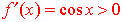 over the intervals (-π/2, π/2), (3π/2, 5π/2), and (7π/2, 9π/2), the function is increasing over those intervals. over the intervals (-π/2, π/2), (3π/2, 5π/2), and (7π/2, 9π/2), the function is increasing over those intervals.
As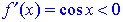 over the intervals (-3π/2, -π/2), (π/2, 3π/2), and (5π/2, 7π/2) the function is decreasing over those intervals. over the intervals (-3π/2, -π/2), (π/2, 3π/2), and (5π/2, 7π/2) the function is decreasing over those intervals.

Exercise 1
Test that the properties stated in the above table are true. You
can examine the fourteen examples provided in the scroll bar on
the top of the applet below or enter your own function in the box
provided. If you enter your own function, you must use the symbols
+ for add, −
for subtract, * for multiply, / for divide, and ^ to raise
to a power. You can also use various mathematical functions: sin,
cos, tan, sec, cot, csc, arcsin, arccos, arctan, exp, ln, log2,
log10, abs, sqrt and cubert. (Here, "abs" is the absolute
value function, "sqrt" is the square root function and
"cubert" is the cube root function.)
Make sure you understand
the following connections between the two graphs.
- When the graph of the function y= f(x) is horizontal then the graph of its derivative y= f '(x) passes through the x axis (is equal to zero). This occurs only at a stationary point.
- When the slope of the function y= f(x) is positive, the graph of its derivative
y= f '(x) is above the x-axis
(is positive).
- When the slope of the function y= f(x) is negative, the graph of its derivative
y= f '(x) is below the x-axis
(is negative).
- When the slope of the function y= f(x) is vertical , the graph of its derivative y= f '(x) is undefined at that value of x.
|
|
Can't see the above java applet? Click here
to see how to enable Java on your web browser. (This applet is based
on free Java applets from JavaMath
)
Exercise 2A
Consider the function f(x) whose graph is shown below.
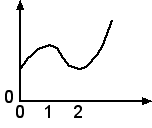 
Click on the graph below
that is f´(x), the derivative of f(x).
 (a) (a)
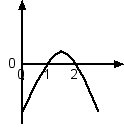 (b) (b)
 (c) (c)
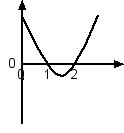 (d) (d)
Example
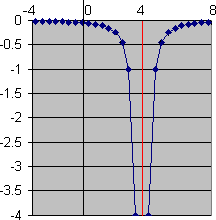
Consider the rational function f(x) =  .
Its graph is a hyperbola with asymptote at x = 4. It is discontinuous
at x = 4. .
Its graph is a hyperbola with asymptote at x = 4. It is discontinuous
at x = 4.

Differentiating, f '(x) =  .
When x = 4, f '(x) = 1/0, which is not defined. All other
values of x have a negative derivative and an associated decreasing slope
on the graph. The graph of the derivative is below. .
When x = 4, f '(x) = 1/0, which is not defined. All other
values of x have a negative derivative and an associated decreasing slope
on the graph. The graph of the derivative is below.
Exercise 2B
Consider
the functions in these 8 exercises.
|

